Accueil

Comment vivre

(Tetractys)

🎲🎲

Cet article est une débauche. Vous pouvez m’aider à l'améliorer.

Mettre en oeuvre la thérapie du rejet :

« La thérapie du rejet » créée par un certain Jason Comely. En fait il s’agit d’un jeu quotidien pour bâtir une solide confiance en soi et vaincre la peur. Ce jeu a déjà conquis des milliers de joueurs aux USA. Les règles sont très simples, il n’y en a qu’une : « Vous devez être rejeté par une autre personne au moins une fois, chaque jour »

Comment vivre > 🎲🎲 10 (Derek:h)

Tout ce qui est bon provient d'une certaine forme de douleur.
La fatigue musculaire vous rend sain et fort.
La douleur de la pratique mène à la maîtrise.
Les conversations difficiles sauvent vos relations.

Mais si vous évitez la douleur, vous évitez l'amélioration.
Si vous évitez l'embarras, vous évitez le succès.
Si vous évitez le risque, vous évitez la récompense.

Tout le monde peut donner le meilleur de lui-même quand tout va bien.
Mais quand les choses vont mal, on voit qui ils sont vraiment.
Rappelez-vous l'arc classique de l'histoire du voyage du héros.
La crise - le moment le plus douloureux - définit le héros.

L'amélioration est une transformation.
Elle apporte la douleur de la perte de l'ancien moi confortable.
Elle apporte la douleur d'une nouvelle série de problèmes.
La richesse apporte la douleur de la responsabilité.
La célébrité apporte la douleur des attentes.
L'amour apporte la douleur de l'attachement.
Si vous évitez la douleur, vous évitez ce que vous voulez vraiment.

Le but de la vie n'est pas le confort.
La recherche du confort est à la fois pathétique et mauvaise pour vous.
Le confort vous rend faible et non préparé.
Si vous vous surprotégez de la douleur, chaque petit défi vous semblera insupportable.
Les gens disent qu'ils ne font pas le travail parce que c'est difficile. Mais c'est difficile parce qu'ils ne font pas le travail.

Le confort est un tueur silencieux.
Le confort est un sable mouvant.
Plus la chaise est molle, plus il est difficile de s'en sortir.

La bonne chose à faire n'est jamais confortable.
La façon dont vous faites face à la douleur détermine qui vous êtes.
Par conséquent, la façon de vivre est de se diriger vers la douleur.
Utilisez-la comme votre boussole.
Toujours prendre l'option la plus difficile.
Toujours pousser dans l'inconfort.
Ignorez vos instincts.

Le pouvoir de la douleur repose sur la surprise.
Si vous l'attendez, elle est plus faible.
Si vous la choisissez, elle disparaît.

Choisir la douleur la rend supportable.
Elle perd son pouvoir de vous blesser.
Vous devenez son maître, pas sa victime.

La douleur arrive de toute façon.
Ne prenez pas de bouclier.
Prends une selle.
Apprivoisez-la.

Ne souhaite pas avoir de la chance.
La chance vous rend complaisant.
Entraîne-toi à prospérer avec la malchance.
La malchance vous rend débrouillard et fort.
Peu importe ce que le monde vous envoie, vous pouvez supporter pire.

Choisir la douleur, c'est aller au-delà de ses instincts.
La nourriture qui a bon goût est mauvaise pour vous, et vice-versa.
Alors n'utilisez pas vos sentiments comme guide.

Choisissez la douleur à petites doses pour renforcer votre résistance à celle-ci.
Un rituel quotidien d'exercice intense donne une excellente perspective sur les autres douleurs de la vie.

Mettez-vous dans des situations stressantes.
Au bout d'un moment, presque rien ne vous semblera stressant.

Socialement, essayez de vous faire rejeter.
Renseignez-vous sur la "thérapie du rejet".**
Faites des demandes audacieuses que vous pensez être refusées.
Cela supprime la douleur du rejet.
Et vous serez surpris de voir combien de fois ils disent oui.

La meilleure façon d'apprendre une langue étrangère est de **cesser de parler sa langue maternelle**.
Peu importe l'embarras ou la frustration, communiquez uniquement dans votre nouvelle langue.
La nécessité est le meilleur professeur.
Mais ça fait mal.

Entraînez-vous à supporter les différents types de douleur.
Tentez quelque chose qui semble impossible - quelque chose qui vous terrifie.
Faites un discours.
Faites une méditation silencieuse de dix jours.
Abandonnez une habitude.
Présentez vos excuses à quelqu'un à qui vous avez fait du tort.

Ne vous félicitez pas si votre tentative évite l'échec.
Rappelez-vous : vous voulez la douleur.
Plus tôt vous payez le prix, moins il coûte cher.

Soyez absolument honnête avec tout le monde.
Arrêtez complètement de mentir.
Tu mens quand tu as peur.
Tu mens pour éviter les conséquences.
Dis toujours la vérité.
Assume les conséquences douloureuses.

Vous n'êtes pas fait pour être oisif.
Tu n'es pas fait pour t'asseoir et regarder des écrans. **
Vous vivez pour pousser, tirer, grimper et grandir.

Les expériences les plus exaltantes de votre vie jusqu'à présent étaient audacieuses. **
Les moments dont vous êtes le plus fier sont ceux où vous avez surmonté un combat.
Le meilleur bonheur vient après une certaine douleur.
Les meilleures vagues sur la plage peuvent vous faire tomber.
C'est le meilleur type de jeu.

Puisque vous ne pouvez pas éviter les problèmes, trouvez de bons problèmes.
Le bonheur n'est pas la tranquillité éternelle.
Le bonheur, c'est de résoudre de bons problèmes.

C'est pourquoi nous jouons à des jeux.
Les jeux sont des défis.
Tout défi peut être transformé en jeu.

Le mot anglais "passion" vient du mot latin "pati", qui signifie "souffrir ou endurer".
Être passionné par quelque chose, c'est être prêt à souffrir pour cette chose, à endurer la douleur qu'elle entraîne.

Mais ne soyez pas masochiste.
Soyez un érudit de la douleur.
Chaque douleur contient une leçon, et une raison pour laquelle elle fait mal.
Analysez-la.
Comprenez-la.

Les fantômes ne partent pas tant que vous n'avez pas compris leur message.
Les problèmes persistent jusqu'à ce que vous les revendiquiez et les résolviez.
Affrontez-les directement et ils disparaîtront.

Nous avons d'abord trouvé comment voler, puis comment aller sur la lune.
Une fois que vous aurez résolu les petits problèmes, vous pourrez affronter les plus importants.

Affronter la douleur t'aide à te rapprocher des autres.
Tes problèmes ne sont jamais uniques.
Quels que soient les problèmes que vous avez eus, beaucoup d'autres personnes ont eu le même problème.
Nous éprouvons de l'empathie pour quelqu'un qui a des difficultés.
Cela nous ouvre davantage le cœur que de voir quelqu'un gagner.

La plupart des gens n'ont pas la possibilité de choisir comment ils souffrent.
Une fois que vous avez apprivoisé la douleur pour vous-même, apprivoisez-la pour les autres.

La voie facile mène à un avenir difficile.
La route difficile mène à un avenir facile.
Se diriger vers la douleur est la façon de vivre.

Carte n°10 à écrire - Gamedesign Comment Vivre - Source Derek > How to live

Everything good comes from some kind of pain.
Muscle fatigue makes you healthy and strong.
The pain of practice leads to mastery.
Difficult conversations save your relationships.

But if you avoid pain, you avoid improvement.
Avoid embarrassment, and you avoid success.
Avoid risk, and you avoid reward.

Anyone can be their best when things are going well.
But when things go wrong, you see who they really are.
Remember the classic story arc of the hero’s journey.
The crisis — the most painful moment — defines the hero.

Improvement is transformation.
It brings the pain of loss of the comfortable previous self.
It brings the pain of a new set of problems.
Wealth brings the pain of responsibility.
Fame brings the pain of expectations.
Love brings the pain of attachment.
If you avoid pain, you avoid what you really want.

The goal of life is not comfort.
Pursuing comfort is both pathetic and bad for you.
Comfort makes you weak and unprepared.
If you overprotect yourself from pain, then every little challenge will feel unbearably difficult.
People say they’re not doing the work because it’s hard. But it’s hard because they’re not doing the work.

Comfort is a silent killer.
Comfort is quicksand.
The softer the chair, the harder it is to get out of it.

The right thing to do is never comfortable.
How you face pain determines who you are.
Therefore, the way to live is to steer towards the pain.
Use it as your compass.
Always take the harder option.
Always push into discomfort.
Ignore your instincts.

Pain’s power relies on surprise.
If you expect it, it’s weaker.
If you choose it, it’s gone.

Choosing pain makes it bearable.
It loses its power to hurt you.
You become its master, not victim.

Pain is coming anyway.
Don’t get a shield.
Get a saddle.
Tame it.

Don’t wish for good luck.
Good luck makes you complacent.
Practice thriving with bad luck.
Bad luck makes you resourceful and strong.
No matter what the world throws your way, you can stand worse.

Choosing pain means pushing past your instincts.
Food that tastes good is bad for you, and vice-versa.
So don’t use your feelings as a guide.

Choose pain in small doses to build your resistance to it.
A daily ritual of hard exercise gives a great perspective on life’s other pains.

Put yourself into stressful situations.
Eventually, almost nothing will seem stressful.

Socially, try to get rejected.

• • Learn about “rejection therapy”.**
    

Make audacious requests that you think will be denied.
This removes the pain of rejection.
And you’ll be surprised how often they say yes.

The best way to learn a foreign language is to **stop speaking your mother tongue.**
No matter how embarrassing or frustrating, communicate only in your new language.
Necessity is the best teacher.
But it hurts.

Practice taking on the various kinds of pain.
Attempt something that seems impossible — something that terrifies you.
Give a speech.
Do a ten-day silent meditation.
Quit a habit.
Apologize to someone you wronged.

Don’t congratulate yourself if your attempt avoids failure.
Remember: you want the pain.
The sooner you pay a price, the less it costs.

Be absolutely honest with everyone.

• • Stop lying, completely.**
    

You lie when you’re afraid.
You lie to avoid consequences.
Always say the truth.
Take the painful consequences.

You weren’t meant to be idle.

• • You weren’t built for sitting and staring at screens. **
    

You live to push, pull, climb, and grow.

• • The most exhilarating experiences in your life so far were daring.**
    

Your proudest moments were overcoming a struggle.
The best happiness comes after some pain.
The best waves on the beach can knock you over.
That’s the best kind of play.

Since you can’t avoid problems, just find good problems.
Happiness isn’t everlasting tranquility.
Happiness is solving good problems.

That’s why we play games.
Games are challenges.
Any challenge can be turned into a game.

The English word “passion” comes from the Latin word “pati”, meaning “to suffer or endure”.
To be passionate about something is to be willing to suffer for it — to endure the pain it’ll bring.

But don’t be a masochist.
Be a scholar of pain.
Every pain has a lesson inside, and a reason why it hurts.
Analyze it.
Understand it.

Ghosts don’t leave until you’ve understood their message.
Problems persist until you claim them and solve them.
Face them directly and they’ll disappear.

First we figured out how to fly, then how to get to the moon.
After you conquer the little problems, you’ll face the better ones.

Facing pain helps you relate to others.
Your problems are never unique.
Whatever problems you’ve had, many other people have had the same problem.
We empathize with someone who’s struggling.
It opens our hearts more than seeing someone win.

Most people don’t get to choose how they suffer.
Once you tame pain for yourself, tame it for others.

The easy road leads to a hard future.
The hard road leads to an easy future.
Steering towards the pain is how to live.

 

This article is about the number. For the years, see 10 BC and AD 10. For other uses, see 10 (disambiguation).

"10th" redirects here. For other uses, see Tenth (disambiguation).

← 9 10 11 →
← 10 11 12 13 14 15 16 17 18 19 → List of numbers Integers ← 0 10 20 30 40 50 60 70 80 90 →
Cardinal	ten
Ordinal	10th (tenth)
Numeral system	decimal
Factorization	2 × 5
Divisors	1, 2, 5, 10
Greek numeral	Ι´
Roman numeral	X
Roman numeral (unicode)	X, x
Greek prefix	deca-/deka-
Latin prefix	deci-
Binary	10102
Ternary	1013
Senary	146
Octal	128
Duodecimal	A12
Hexadecimal	A16
Chinese numeral	十，拾
Hebrew	י (Yod)
Khmer	១០
Armenian	Ժ
Tamil	௰
Thai	๑๐
Devanāgarī	१०
Bengali	১০
Arabic & Kurdish & Iranian	١٠
Malayalam	൰
Egyptian hieroglyph	𓎆
Babylonian numeral	𒌋

10 (ten) is the even natural number following 9 and preceding 11. Ten is the base of the decimal numeral system, the most common system of denoting numbers in both spoken and written language.

Anthropology

Usage and terms

• A collection of ten items (most often ten years) is called a decade.
• The ordinal adjective is decimal; the distributive adjective is denary.
• Increasing a quantity by one order of magnitude is most widely understood to mean multiplying the quantity by ten.
• To reduce something by one tenth is to decimate. (In ancient Rome, the killing of one in ten soldiers in a cohort was the punishment for cowardice or mutiny; or, one-tenth of the able-bodied men in a village as a form of retribution, thus causing a labor shortage and threat of starvation in agrarian societies.)

Mathematics

Ten is the fifth composite number, and the smallest noncototient, which is a number that cannot be expressed as the difference between any integer and the total number of coprimes below it. Ten is the eighth Perrin number, preceded by 5, 5, and 7.

As important sums,

• $10=1^{2}+3^{2}$, the sum of the squares of the first two odd numbers
• $10=1+2+3+4$, the sum of the first four positive integers, equivalently the fourth triangle number
• $10=3+7=5+5$, the smallest number that can be written as the sum of two prime numbers in two different ways
• $10=2+3+5$, the sum of the first three prime numbers, and the smallest semiprime that is the sum of all the distinct prime numbers from its lower factor through its higher factor

The factorial of ten is equal to the product of the factorials of the first four odd numbers as well: $10!=1!\cdot 3!\cdot 5!\cdot 7!$, and 10 is the only number whose sum and difference of its prime divisors yield prime numbers $(2+5=7$ and $5-2=3)$.

10 is also the first number whose fourth power (10,000) can be written as a sum of two squares in two different ways, $80^{2}+60^{2}$ and $96^{2}+28^{2}.$

Ten has an aliquot sum of 8, and is the first discrete semiprime $(2\times 5)$ to be in deficit, as with all subsequent discrete semiprimes. It is the second composite in the aliquot sequence for ten (10, 8, 7, 1, 0) that is rooted in the prime 7-aliquot tree.

It is a largely composite number, as it has 4 divisors and no smaller number has more than 4 divisors.

According to conjecture, ten is the average sum of the proper divisors of the natural numbers $$

 
   
     
       
         N
       
     
   
   {\displaystyle \mathbb {N} }
 

$\mathbb {N}$ if the size of the numbers approaches infinity, and it is the smallest number whose status as a possible friendly number is unknown.

The smallest integer with exactly ten divisors is 48, while the least integer with exactly eleven divisors is 1024, which sets a new record.

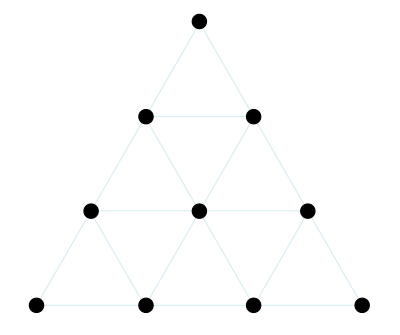
Figurate numbers that represent regular ten-sided polygons are called decagonal and centered decagonal numbers. On the other hand, 10 is the first non-trivial centered triangular number and tetrahedral number. 10 is also the first member in the coordination sequence for body-centered tetragonal lattices.

While 55 is the tenth triangular number, it is also the tenth Fibonacci number, and the largest such number to also be a triangular number. 55 is also the fourth doubly triangular number.

10 is the fourth telephone number, and the number of Young tableaux with four cells. It is also the number of $n$-queens problem solutions for $n=5$.

There are precisely ten small Pisot numbers that do not exceed the golden ratio.

Geometry

Decagon

Main article: Decagon

As a constructible polygon with a compass and straight-edge, the regular decagon has an internal angle of $12^{2}=144$ degrees and a central angle of $6^{2}=36$ degrees. All regular $n$-sided polygons with up to ten sides are able to tile a plane-vertex alongside other regular polygons alone; the first regular polygon unable to do so is the eleven-sided hendecagon. While the regular decagon cannot tile alongside other regular figures, ten of the eleven regular and semiregular tilings of the plane are Wythoffian (the elongated triangular tiling is the only exception); however, the plane can be covered using overlapping decagons, and is equivalent to the Penrose P2 tiling when it is decomposed into kites and rhombi that are proportioned in golden ratio. The regular decagon is also the Petrie polygon of the regular dodecahedron and icosahedron, and it is the largest face that an Archimedean solid can contain, as with the truncated dodecahedron and icosidodecahedron.

There are ten regular star polychora in the fourth dimension, all of which have orthographic projections in the $$

 
   
     
       
         
           H
         
         
           3
         
       
     
   
   {\displaystyle \mathrm {H} _{3}}
 

$\mathrm {H} _{3}$ Coxeter plane that contain various decagrammic symmetries, which include compound forms of the regular decagram.

Higher-dimensional spaces

$\mathrm {M} _{10}$ is a multiply transitive permutation group on ten points. It is an almost simple group, of order,

$720=2^{4}\cdot 3^{2}\cdot 5=2\cdot 3\cdot 4\cdot 5\cdot 6=8\cdot 9\cdot 10$

It functions as a point stabilizer of degree 11 inside the smallest sporadic simple group $\mathrm {M} _{11}$, a group with an irreducible faithful complex representation in ten dimensions, and an order equal to  $7920=11\cdot 10\cdot 9\cdot 8$  that is one more than the one-thousandth prime number, 7919.

$$

 
   
     
       
         
           E
         
         
           10
         
       
     
   
   {\displaystyle \mathrm {E} _{10}}
 

$\mathrm {E} _{10}$ is an infinite-dimensional Kac–Moody algebra which has the even Lorentzian unimodular lattice II_9,1 of dimension 10 as its root lattice. It is the first $$

 
   
     
       
         
           E
         
         
           n
         
       
     
   
   {\displaystyle \mathrm {E} _{n}}
 

$\mathrm {E} _{n}$ Lie algebra with a negative Cartan matrix determinant, of −1.

There are precisely ten affine Coxeter groups that admit a formal description of reflections across $$

 
   
     
       n
     
   
   {\displaystyle n}
 

$n$ dimensions in Euclidean space. These contain infinite facets whose quotient group of their normal abelian subgroups is finite. They include the one-dimensional Coxeter group $$

 
   
     
       
         
           
             
               I
               ~
             
           
         
         
           1
         
       
     
   
   {\displaystyle {\tilde {I}}_{1}}
 

${\tilde {I}}_{1}$ [∞], which represents the apeirogonal tiling, as well as the five affine Coxeter groups $$

 
   
     
       
         
           
             
               G
               ~
             
           
         
         
           2
         
       
     
   
   {\displaystyle {\tilde {G}}_{2}}
 

${\tilde {G}}_{2}$, $$

 
   
     
       
         
           
             
               F
               ~
             
           
         
         
           4
         
       
     
   
   {\displaystyle {\tilde {F}}_{4}}
 

${\tilde {F}}_{4}$, $$

 
   
     
       
         
           
             
               E
               ~
             
           
         
         
           6
         
       
     
   
   {\displaystyle {\tilde {E}}_{6}}
 

${\tilde {E}}_{6}$, $$

 
   
     
       
         
           
             
               E
               ~
             
           
         
         
           7
         
       
     
   
   {\displaystyle {\tilde {E}}_{7}}
 

${\tilde {E}}_{7}$, and $$

 
   
     
       
         
           
             
               E
               ~
             
           
         
         
           8
         
       
     
   
   {\displaystyle {\tilde {E}}_{8}}
 

${\tilde {E}}_{8}$ that are associated with the five exceptional Lie algebras. They also include the four general affine Coxeter groups $$

 
   
     
       
         
           
             
               A
               ~
             
           
         
         
           n
         
       
     
   
   {\displaystyle {\tilde {A}}_{n}}
 

${\tilde {A}}_{n}$, $$

 
   
     
       
         
           
             
               B
               ~
             
           
         
         
           n
         
       
     
   
   {\displaystyle {\tilde {B}}_{n}}
 

${\tilde {B}}_{n}$, $$

 
   
     
       
         
           
             
               C
               ~
             
           
         
         
           n
         
       
     
   
   {\displaystyle {\tilde {C}}_{n}}
 

${\tilde {C}}_{n}$, and $$

 
   
     
       
         
           
             
               D
               ~
             
           
         
         
           n
         
       
     
   
   {\displaystyle {\tilde {D}}_{n}}
 

${\tilde {D}}_{n}$ that are associated with simplex, cubic and demihypercubic honeycombs, or tessellations. Regarding Coxeter groups in hyperbolic space, there are infinitely many such groups; however, ten is the highest rank for paracompact hyperbolic solutions, with a representation in nine dimensions. There also exist hyperbolic Lorentzian cocompact groups where removing any permutation of two nodes in its Coxeter–Dynkin diagram leaves a finite or Euclidean graph. The tenth dimension is the highest dimensional representation for such solutions, which share a root symmetry in eleven dimensions. These are of particular interest in M-theory of string theory.

Science

The SI prefix for 10 is "deca-".

The meaning "10" is part of the following terms:

• decapoda, an order of crustaceans with ten feet.
• decane, a hydrocarbon with 10 carbon atoms.

Also, the number 10 plays a role in the following:

• The atomic number of neon.
• The number of hydrogen atoms in butane, a hydrocarbon.
• The number of spacetime dimensions in some superstring theories.

The metric system is based on the number 10, so converting units is done by adding or removing zeros (e.g. 1 centimetre = 10 millimetres, 1 decimetre = 10 centimetres, 1 meter = 100 centimetres, 1 dekametre = 10 meters, 1 kilometre = 1,000 meters).

Music

See also: Ten (disambiguation) § Art and entertainment

• The interval of a major tenth is an octave plus a major third.
• The interval of a minor tenth is an octave plus a minor third.

Religion

The tetractys

Abrahamic religions

The Ten Commandments in the Hebrew Bible are ethical commandments decreed by God (to Moses) for the people of Israel to follow.

Mysticism

• In Pythagoreanism, the number 10 played an important role and was symbolized by the tetractys.

• There are Ten Sephirot in the Kabbalistic Tree of Life.

• In Chinese astrology, the 10 Heavenly Stems, refer to a cyclic number system that is used also for time reckoning.

See also

• Mathematics portal
• List of highways numbered 10

Photothèque

• Essai de l'application Glass